NCERT Class 7 Mathematics Textbook for blind and low vision students made Screen Readable by Professor T K Bansal.

We can add and subtract integers. Let us now learn how to multiply integers.

1.4.1 Multiplication of a Positive and a Negative Integer

We know that multiplication of whole numbers is repeated addition.

For example,
5 + 5 + 5 = 3 × 5 = 15

Can you represent addition of integers in the same way?

TRY THESE 1.4

Find:
4 × (− 8),
8 × (−2),
3 × (−7),
10 × (−1)
using number line.

We have from the following number line, (−5) + (−5) + (−5) = −15

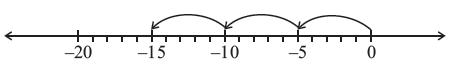
But we can also write
(−5) + (−5) + (−5) = 3 × (−5)

Therefore, 3 × (−5) = −15
Similarly ( −4) + ( −4) + ( −4) + ( −4) + ( −4) = 5 × ( −4) = −20

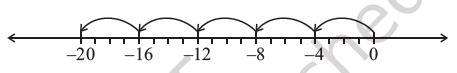
And ( −3) + ( −3) + ( −3) + ( −3) = __________ = __________
Also, ( −7) + ( −7) + ( −7) = __________ = __________

Let us see how to find the product of a positive integer and a negative integer without using number line.

Let us find 3 × (−5) in a different way.
First find 3 × 5 and then put minus sign (−) before the product obtained. You get −15. That is we find − (3 × 5) to get −15.

Similarly, 5 × (− 4) = − (5×4) = − 20.

Find in a similar way,
4 × (− 8) = _____ = _____,
3 × ( −7) = _____ = _____
6 × ( −5) = _____ = _____,
2 × ( −9) = _____ = _____

Using this method we thus have,
10 × ( −43) = _____ = − (10 × 43) = − 430

Till now we multiplied integers as (positive integer) × (negative integer).

Let us now multiply them as (negative integer) × (positive integer).
We first find −3 × 5.

To find this, observe the following pattern:
We have, 3 × 5 = 15
2 × 5 = 10 = 15 − 5
1 × 5 = 5 = 10 − 5
0 × 5 = 0 = 5 − 5

So, −1 × 5 = 0 − 5 = −5
−2 × 5 = −5 − 5 = −10
−3 × 5 = −10 − 5 = −15

We already have 3 × ( −5) = −15
So we get ( −3) × 5 = −15 = 3 × ( −5)

Using such patterns, we also get (−5) × 4 = −20 = 5 × (− 4)
Using patterns, find
( −4) × 8,
( −3) × 7,
( −6) × 5 and
( −2) × 9

Check whether,
( −4) × 8 = 4 × ( −8),
( −3) × 7 = 3 × ( −7),
( −6) × 5 = 6 × ( −5)
and ( −2) × 9 = 2 × ( −9) ?

Using this we get, ( −33) × 5 = 33 × ( −5) = −165.

TRY THESE 1.5

Find:
(i) 6 × ( −19)
(ii) 12 × ( −32)
(iii) 7 × ( −22).

We thus find that while multiplying a positive integer and a negative integer,
We multiply them as whole numbers and put a minus sign (−) before the product.
We thus get a negative integer.

TRY THESE 1.6

Q1. Find:
(a) 15 × (−16)
(b) 21 × (−32)
(c) (− 42) × 12 (d) −55 × 15

Q2. Check if
(a) 25 × (−21) = (−25) × 21
(b) ( −23) × 20 = 23 × ( −20)
Write five more such examples.

In general, for any two positive integers a and b we can say
a × (− b) = (− a) × b = − (a × b)

1.4.2 Multiplication of two Negative Integers

Can you find the product ( −3) × ( −2)?

Observe the following:
−3 × 4 = − 12
−3 × 3 = −9 = −12 − (−3)
−3 × 2 = − 6 = −9 − (−3)
−3 × 1 = −3 = − 6 − (−3)
−3 × 0 = 0 = −3 − (−3)
−3 × −1 = 0 − (−3) = 0 + 3 = 3
−3 × −2 = 3 − (−3) = 3 + 3 = 6

Do you see any pattern? Observe how the products change.

Based on this observation, complete the following:
−3 × −3 = _____ −3 × − 4 = _____

Now observe these products and fill in the blanks:
− 4 × 4 = −16
− 4 × 3 = −12 = −16 + 4
− 4 × 2 = _____ = −12 + 4
− 4 × 1 = _____
− 4 × 0 = _____
− 4 × (−1) = _____
− 4 × (−2) = _____
− 4 × (−3) = _____

From these patterns we observe that,
(−3) × (−1) = 3 = 3 × 1
(−3) × (−2) = 6 = 3 × 2
(−3) × (−3) = 9 = 3 × 3
and (− 4) × (−1) = 4 = 4 × 1
So, (− 4) × (−2) = 4 × 2 = _____
(− 4) × (−3) = _____ = _____

TRY THESE 1.7

(i) Starting from ( −5) × 4, find ( −5) × (− 6)
(ii) Starting from ( −6) × 3, find ( −6) × ( −7)

So observing these products we can say that the product of two negative integers is a positive integer.
We multiply the two negative integers as whole numbers and put the positive sign before the product.

Thus, we have ( −10) × ( −12) = + 120 = 120
Similarly ( −15) × ( −6) = + 90 = 90

In general, for any two positive integers a and b,
(− a) × (− b) = a × b

TRY THESE 1.8

Find:
( −31) × ( −100),
( −25) × ( −72),
( −83) × ( −28)

Game 1

(i) Take a board marked from −104 to 104 as shown in the table.

71

104	103	102	101	100	99	98	97	96	95	94
83	84	85	86	87	88	89	90	91	92	93 /td>
82	81	80	79	78	77	76	75	74	73	72
61	62	63	64	65	66	67	68	69	70
60	59	58	57	56	55	54	53	52	51	50
39	40	41	42	43	44	45	46	47	48	49
38	37	36	35	34	33	32	31	30	29	28
17	18	19	20	21	22	23	24	25	26	27
16	15	14	13	12	11	10	9	8	7	6
−5	−4	−3	−2	−1	0	1	2	3	4	5
−6	−7	−8	−9	−10	−11	−12	−13	−14	−15	−16
−27	−26	−25	−24	−23	−22	−21	−20	−19	−18	−17
−28	−29	−30	−31	−32	−33	−34	−35	−36	−37	−38
−49	−48	−47	−46	−45	−44	−43	−42	−41	−40	−39
−50	−51	−52	−53	−54	−55	−56	−57	−58	−59	−60
−71	−70	−69	−68	−67	−66	−65	−64	−63	−62	−61
−72	−73	−74	−75	−76	−77	−78	−79	−80	−81	−82
−93	−92	−91	−90	−89	−88	−87	−86	−85	−84	−83
−94	−95	−96	−97	−98	−99	−100	−101	−102	−103	−104

(ii) Take a bag containing two blue and two red dice. Number of dots on the blue dice indicate positive integers and number of dots on the red dice indicate negative integers.

(iii) Every player will place his/her counter at zero.

(iv) Each player will take out two dice at a time from the bag and throw them.

(v) After every throw, the player has to multiply the numbers marked on the dice.

(vi) If the product is a positive integer then the player will move his counter towards 104; if the product is a negative integer then the player will move his counter towards −104.

(vii) The player who reaches either −104 or 104 first is the winner.

(v) After every throw, the player has to multiply the numbers marked on the dice.

(vi) If the product is a positive integer then the player will move his counter towards 104; if the product is a negative integer then the player will move his counter towards −104.

(vii) The player who reaches either −104 or 104 first is the winner.

1.4.3 Product of three or more Negative Integers

Euler in his book Ankitung zur Algebra(1770), was one of the first mathematicians to attempt to prove (−1) × (−1) = 1

We observed that the product of two negative integers is a positive integer.

What will be the product of three negative integers? Four negative integers? .. ..

Let us observe the following examples:
(a) ( −4) × ( −3) = 12
(b) ( −4) × ( −3) × ( −2) = [( −4) × ( −3)] × ( −2) = 12 × ( −2) = − 24
(c) ( −4) × ( −3) × ( −2) × ( −1) = [( − 4) × ( −3) × ( −2)] × ( −1) = ( −24) × ( −1)
(d) ( −5) × [( −4) × ( −3) × ( −2) × ( −1)] = ( −5) × 24 = −120

A Special Case

Consider the following statements and the resultant products:
(−1) × (−1) = +1
(−1) × (−1) × (−1) = −1
(−1) × (−1) × (−1) × (−1) = +1
(−1) × (−1) × (−1) × (−1) × (−1) = −1

This means that if the integer (−1) is multiplied even number of times, the product is +1 and if the integer (−1) is multiplied odd number of times, the product is −1.
You can check this by making pairs of ( −1) in the statement.
This is useful in working out products of integers.

From the above products we observe that
(a) the product of two negative integers is a positive integer;
(b) the product of three negative integers is a negative integer.
(c) product of four negative integers is a positive integer.
What is the product of five negative integers in (d)?

So what will be the product of six negative integers?

We further see that in (a) and (c) above, the number of negative integers that are multiplied are even [two and four respectively] and the product obtained in (a) and (c) are positive integers. The number of negative integers that are multiplied in (b) and (d) is odd and the products obtained in (b) and (d) are negative integers.

We find that if the number of negative integers in a product is even, then the product is a positive integer; if the number of negative integers in a product is odd, then the product is a negative integer.

Justify it by taking five more examples of each kind.

THINK, DISCUSS AND WRITE

(i) The product ( −9) × ( −5) × ( −6) × ( −3) is positive whereas the product ( −9) × ( −5) × 6 × ( −3) is negative. Why?

(ii) What will be the sign of the product if we multiply together:
(a) 8 negative integers and 3 positive integers?
(b) 5 negative integers and 4 positive integers?
(c) ( −1), twelve times?
(d) ( −1), 2m times, m is a natural number?